[php snippet=1]
The cruising altitude of most planes is about 10,000 meters above sea level where there is less air above the plane pushing down, so the air pressure is lower (about 20 kPa outside compared to about 100 kPa atmospheric pressure at sea level). To keep everyone comfortable inside the plane, the cabin is pressurized to about 75 kPa. The passenger doors on a 747 are about 1 meter wide by 2 meters tall.
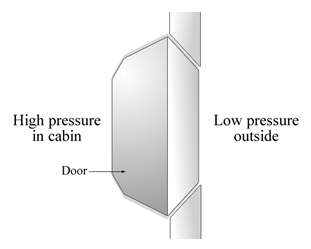
If a metric ton is 1000 kg, to pull the door open at 10,000 meters is equivalent to moving how many metric tons? (the acceleration due to gravity can be approximated as 10 m/s2)

Loading ...
Correct Answer: D. 11 tons.
This is the kind of reasoning question that you can expect as a result of an MCAT passage.
The difference in pressure between inside and outside the cabin at 10,000 meters is:
75 – 20 = 55 kPa = 55,000 Pa or N/m2 of pressure pushing on the door (keep in mind that the SI unit for pressure is the pascal, Pa).
Pressure is defined as force per unit area: P = F/A
The area of the door is 2 x 1 = 2m2
So F = PA = (55,000)(2) = 110,000 N
Thus weight mg = 110,000, and since g = 10 m/s2, then m = 11,000 kg or 11 metric tons.
Solving this problem requires reasoning, dimensional analysis and one very basic equation. List of physics equations to memorize: www.goldstandard-mcat.com/physics-equation-lists/
Today’s question has been brought to you by Gold Standard MCAT.
Get more passage-based questions at Gold Standard MCAT.
Subscribe below to receive the MCAT Question of the Day delivered straight to your inbox every morning.










